
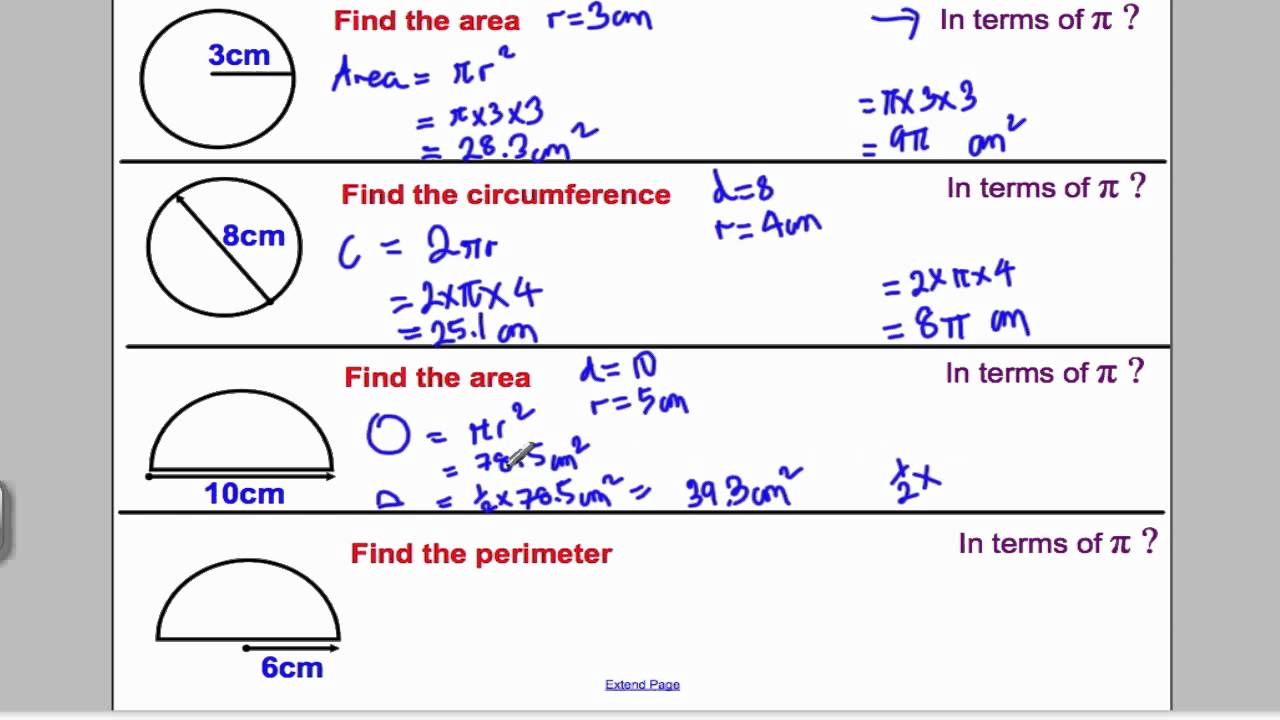

Given that angle ADB, which is 69\degree, is the angle between the side of the triangle and the tangent, then the alternate segment theorem immediately gives us that the opposite interior angle, angle AED (the one we’re looking for), is also 69\degree. It would seem as though for each one we take the number of times (it occurs) / the number of objects there are. This tells us that the angle between the tangent and the side of the triangle is equal to the opposite interior angle. Now we can use our second circle theorem, this time the alternate segment theorem. 12 4 Practice B Mathbitsnotebook geometry ccss lessons and practice. Let the size of one of these angles be x, then using the fact that angles in a triangle add to 180, we get circle independent practice answer key Stop my calculator showing fractions as answers. In this case those two angles are angles BAD and ADB, neither of which know.
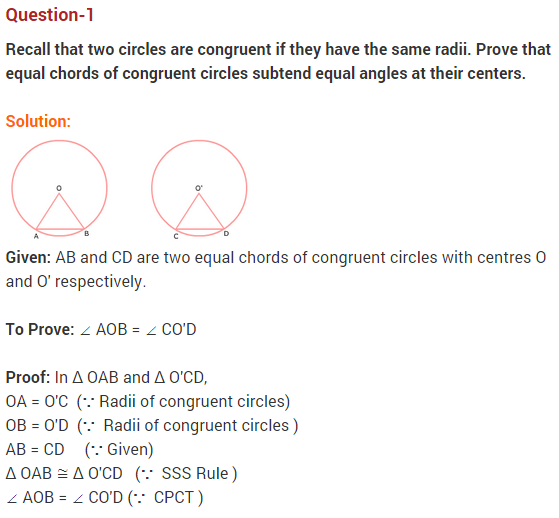
This means that ABD must be an isosceles triangle, and so the two angles at the base must be equal. Advertisement AdvertisementUnit 10: Circles Homework 5: Tangent Lines Quiz problems. Our first circle theorem here will be: tangents to a circle from the same point are equal, which in this case tells us that AB and BD are equal in length. Answers : Gebhard Curt Geometry Unit 9 Circles from lh5.


 0 kommentar(er)
0 kommentar(er)
